本文最后更新于358 天前,其中的信息可能已经过时,如有错误请发送邮件到727189340@qq.com
Kruskal 算法
原理
Kruskal 算法由两个步骤构成:
- 对边的长度贪心并加入 $T$ 中。先对边长排序,然后依次把最短的边加入到 $T$ 中。
- 判断圈。每次加入边,判断它是否和已经加入 $T$ 的边形成了圈,也就是判断连通性。可以使用 DFS 或者 BFS,但是最高效的方法是 并查集。
模板代码
下面的代码作为最小生成树的模板题,解决了P3366 【模板】最小生成树问题。
#include <iostream>
#include <algorithm>
using namespace std;
int read()
{
int x = 0, f = 1;
char ch = 0;
while (!isdigit(ch))
{
if (ch == '-')
f = -1;
ch = getchar();
}
while (isdigit(ch))
{
x = x * 10 + ch - '0';
ch = getchar();
}
return x * f;
}
const int maxN = 5005;
const int maxM = 2e5 + 5;
struct Edge
{
int u, v, w;
} edge[maxM];
int s[maxN]; // 并查集
bool cmp(Edge a, Edge b)
{
return a.w < b.w;
}
// 并查集 - 查询
int find_set(int x)
{
if (x != s[x])
s[x] = find_set(s[x]);
return s[x];
}
int N, M;
void kruskal()
{
sort(edge + 1, edge + M + 1, cmp);
for (int i = 1; i <= N; i++)
s[i] = i;
int ans = 0, cnt = 0; // cnt 存储已经放入 T 中的边数
for (int i = 1; i <= M; i++)
{
if (cnt == N - 1)
break;
int e1 = find_set(edge[i].u);
int e2 = find_set(edge[i].v);
if (e1 == e2)
continue;
else
{
ans += edge[i].w;
s[e2] = e1;
cnt++;
}
}
if (cnt == N - 1)
printf("%d\n", ans);
else
printf("orz");
}
int main()
{
N = read();
M = read();
for (int i = 1; i <= M; i++)
{
edge[i].u = read();
edge[i].v = read();
edge[i].w = read();
}
kruskal();
return 0;
}Prim 算法
执行过程
设最小生成树中的点的集合为 $U$,开始时最小生成树为空,所以 $U$ 为空。以下图为例,执行步骤如下:
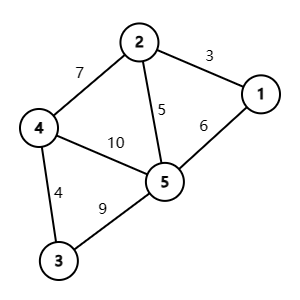
- 任取一点,如点 $1$,放到 $U$ 中,$U = {1}$。
- 找离集合中的点最近的邻居,即点 $1$ 的邻居,为点$2$,放到 $U$ 中,$U = {1,2}$。
- 找离 $2$ 最近的点,为 $5$,放入 $U$ 中,$U = {1,2,5}$
- 离 $5$ 最近的此时是 $1$(上一步已经构造了 $2 -5$,所以不考虑那一条边),但 $1$ 已经在集合中了,我们就选择 $4$。
- 以此类推,直到所有点都在 $U$ 中。
观察发现,Prim 算法的思想和 Dijkstra 算法基本相同。但是 Dijkstra 算法需要更新 $U$ 中所有点到起点的距离,Prim 算法不需要。同理地,用优先队列优化 Prim 算法能最终将复杂度降低至 $O(m\log_2n)$。
模板代码
#include <iostream>
#include <vector>
#include <queue>
using namespace std;
int read()
{
int x = 0, f = 1;
char ch = 0;
while (!isdigit(ch))
{
if (ch == '-')
f = -1;
ch = getchar();
}
while (isdigit(ch))
{
x = x * 10 + ch - '0';
ch = getchar();
}
return x * f;
}
const int maxN = 5005;
const int maxM = 2e5 + 5;
struct edge
{
int to, w;
edge(int a, int b) { to = a, w = b; }
};
struct node
{
int id, dis;
node(int a, int b) { id = a, dis = b; }
bool operator<(const node &u) const { return dis > u.dis; }
};
int n, m;
bool done[maxN];
vector<edge> G[maxM];
void prim(int n)
{
int s = 1;
for (int i = 1; i <= n; i++)
done[i] = false;
priority_queue<node> q;
q.push({node(s, 0)}); // 从 s 点开始处理数据
int ans = 0, cnt = 0;
while (!q.empty())
{
node u = q.top();
q.pop();
if (done[u.id])
continue;
done[u.id] = true;
ans += u.dis;
cnt++;
for (int i = 0; i < G[u.id].size(); i++)
{
edge y = G[u.id][i];
if (done[y.to])
continue;
q.push({y.to, y.w});
}
}
if (cnt == n)
printf("%d\n", ans);
else
printf("orz");
}
int main()
{
n = read();
m = read();
for (int i = 1; i <= m; i++)
{
int a, b, w;
a = read();
b = read();
w = read();
G[a].push_back(edge(b, w));
G[b].push_back(edge(a, w));
}
prim(n);
return 0;
}